Les seccions còniques:
Circumferència, el·lipse, paràbola i hipèrbola.
This artisan cone produced by Francisco Treceño's workshop consists of five pieces. This shows curves called conical sections:
- The circumference. It is obtained when the cutting plane is perpendicular to the cone axis, it is considered a special case of ellipse.
- The ellipse. It is achieved when the section between the cutting plane and the conical surface is a closed curve.
- The parabola, which is obtained when the cutting plan is parallel to the cone generating. It's an open curve.
- Hyperbola, which is achieved when the plane cuts to both parts of the conical surface. It doesn't necessarily have to be parallel to the cone axis. The resulting section, hyperbola, consists of two open symmetrical branches.
- Hotel Area: Sala Pere Puig Adam
- Minimum age: from 6 years old.
- Required time: 5 minutes.
- Number of participants: One or more people
- Keywords: circle, ellipse, parabola, hyperbola, conical surface
- Taxonomy: GEOMETRY

Construction of a model of the conical sections.
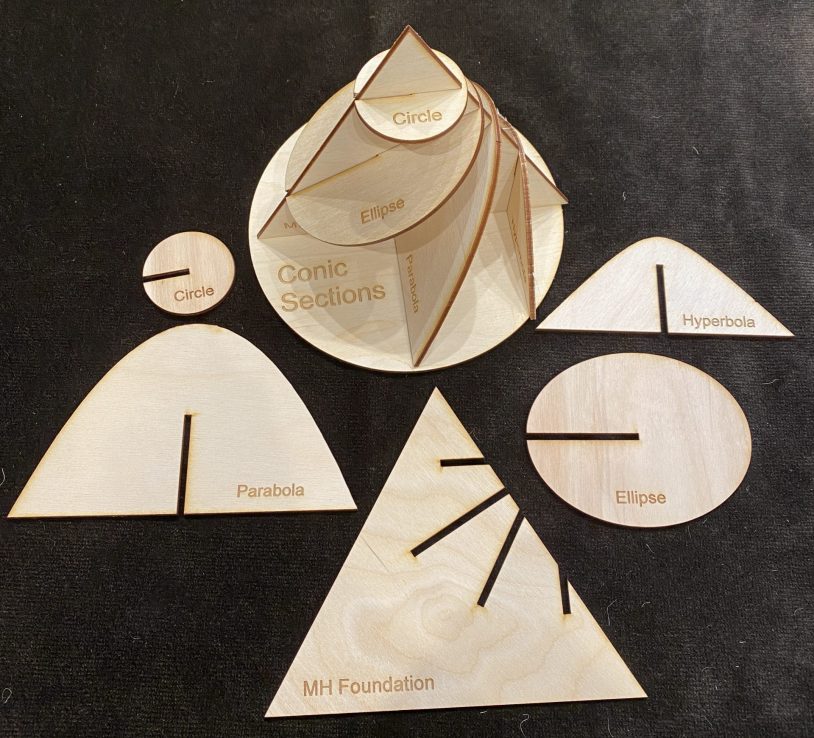
Following in the footsteps of our MathHappens friends, we propose the construction of your own model of conical sections. It consists of 6 pieces, the 4 conical, the circular base and the vertical support triangle.
You can download here the files with the different manufacturing options offered by MathHappens. You can also go directly to their page.
Apollonius of Perge and the conical sections.
Apollonius of Perge was one of the great mathematicians of classical Greece, living in Alexandria, Pergamon and Ephesians. He wrote his main work "Conics" around 200 BC. Giving name to these curves and systematizing the previous knowledge scattered.
Hypacia, Kepler and conics as trajectories of the planets.
In the 2009 film AGORA that recreates the story of the astronomer Hypatia in first-century Alexandria, Apollonius' cone appears. In one scene, Hypatia relates the trajectories of the planets to conics, this is a cinematic license, Hypatia knew about Apoloni's works, but we are not aware that she had established her relationship with planetary orbits.
It was not until the seventeenth century that Kepler recovered these curves from Apollonius' writings stating that celestial trajectories were not the complicated epicycles (spheres rotating on spheres) but the simple ellipses.
