
The birth of the first urban cultures.
Mesopotamia is the region of western Asia between the Tigris and Euphrates Rivers in present-day Iraq. Due to its geographical position, the crossroads between the Red Sea, the Mediterranean, Black, the Caspia, and the Persian Gulf, and its agricultural wealth, is where the first cities-states emerged from the 3rd millennium BC.
We know these civilizations well thanks to the fact that we have managed to decipher their writing. It is the cuneiform writing consisting of incisions or marks on clay tablets that were easily obtained by sinking a stick over soft mud. These writings have endured over time over other systems such as the Egyptian papyrus or wooden marks of other civilizations. They have allowed us to know very well their daily life, their laws, legends and customs.
Approximately one million tablets have been unearthed, a few hundred have numerical content. They deal with administrative, business, mathematics and astronomy records.
The Babylonian numbering system
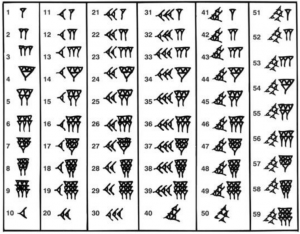
The Babylonian numbering system is, like ours, a positional system. This means that the same symbol has different values depending on the position it occupies, for example in the 342427 2 means once 2000 and the other 20. It is a much more powerful system compared to the Roman numbering system that will be used later where its symbols, I, V, X, D, M have the same value regardless of their position.
Mesopotamians used two types of marks:
- The nail, which is a thin, vertical incision that represents the unit.
- The spike, a triangle-shaped incision that had a value of 10 units
With these two signs, the numbers were represented additively up to 59
For larger quantities they used the same thing we still use with hours, minutes and seconds. A positional system based on 60

The Babylonian Tablet YBC 7289
This reproduction, which you can find in our museum's shop, maintains the same size and material, from the original tablet unearthed in Mesopotamia. It is currently part of the yale university collection.
The tablet is considered to have been made by a student who lived between 1800 and 1600 BC.
It contains an approximation, exceptional for its age and precision, of the diagonal of a square and, therefore, of the root of 2
This way we can read the marks of the YBC 7289 tablet located on the drawing of a square. It is observed that one side of the square is marked with the 30, it turns out that the diagonal of a square of 30 units on the side is approximately 42, an amount that also appears. This already indicates that the marks are square measurements.
The bottom 3 numbers can be read as 42 hours, 25 minutes and 35 seconds, which is a simplified way of saying 42 units, 25 sixty parts of the unit and 35 three hundred-sixty parts of the unit. Numerically it is
42 + 25/60 + 35/(60*60) = 42.426388888...
which we can compare with the result obtained using the Pythagorean Theorem
30*Root 2=42.42640687... A difference of a ten-thousandth!
Doing the same with the 4 core values interpreted as 1 hour 24 minutes 51 seconds and 10 sixty parts of a second.
1 + 24/60 + 51/(60*60) + 10/(60*60*60) = 1.41421296296...
Which corresponds to the diagonal value of a square on side 1 and numerically we can compare it with the calculation using Pythagoras: root 2=1.4142135624...
An impressive approximation, accurate to the sixth decimal place, a thousand years before Pythagoras.
The Mesopotamians did not formalize this theorem, but they used it perfectly. In addition, the accuracy of the measurement shows that they had a numerical method, which we do not know, to obtain this result.
To find out more
- PLA, AND CARRERA, Josep (2016). History of mathematics: Egypt and Mesopotamia. Barcelona. IEC. ISBN: 978-84-9965-308-2
- KRAMER, Samuel Noah (1956). History begins in Sumer. Barcelona 1958. Discontinued but can be found with an internet search. He explains the life of Mesopotamian societies from his texts.
- NUMBERPHILE. Cuneiform Numbers. https://youtu.be/RR3zzQP3bII. It explains, among other things, how to make numerical cuneiform marks using pastelin and a stick of ice cream.
- ELEANOR ROBSON, this professor at the University of Cambridge, has prepared this interesting activity for high school students https://motivate.maths.org/content/BabylonianMaths/
