Put the faces of the polyhedra so that the colors of the faces in contact match
- Location: Martin Gardner Room
- Minimum age: from 6 years old.
- Required time: 5 minutes each
- Number of participants: One or more people
- Keywords: permutations, cube, dodecahedron
- Taxonomy: Geometry, Combinatorics

Color matching is at vertices

The color match is on the edges
How to combine colors on faces?
El cas del cub
In the case of the cube, it turns out that the circular permutations of 4 elements are precisely 6. You can put the 6 faces on the table with a color always on the top to see that there is indeed no different way of these 6 to order the 4 colors.

It coincides that the cube also has six faces and that it is possible to reconstruct it with these six squares so that the colors match.
Once resolved, look at: What are the opposite faces like?
El cas del dodecaedre
En el cas del dodecaedre, les permutacions circulars de 5 elements són 24.
Podem sempre fixar un dels 5 colors en una posició. Ens queden 4 colors per combinar de totes les maneres possibles en les altres posicions, això són les permutacions de 4 elements que es calculen amb la clàssica fórmula de factorial de 4 4! = 4·3·2·1 = 24
Com que el dodecaedre té 12 cares, sols utilitzem la meitat de les cares possibles.
By working methodically in an orderly manner it is possible to reconstruct the dodecahedron in a few minutes. Once resolved notice how the opposite faces are symmetrical. Thus, the 12 chosen faces can each be paired with their symmetry.
En matemàtiques anomenem transposició al moviment consistent en intercanviar dos elements d’un conjunt ordenat. Sempre es pot passar d’una ordenació a una altra fent les transposicions que calgui.
Agafa dues cares qualssevol, pensa: Quines parelles de colors cal transposar per passar d’una cara a l’altra? Quantes transposicions has fet?
Comprovaràs que sempre ens han estat necessaris fer un nombre parell de transposicions. No trobaràs dues cares que es distingeixin sols per tenir dos colors intercanviats. Això és un resultat de la teoria matemàtica de grups!
El conjunt de les 24 cares amb totes les permutacions circulars de 5 colors s’anomena en matemàtiques grup simètric S4 . Té 12 elements amb paritat parella (l’element de referència i els que s’obtenen d’ell amb un nombre parell de transposicions) i 12 elements de paritat senar.
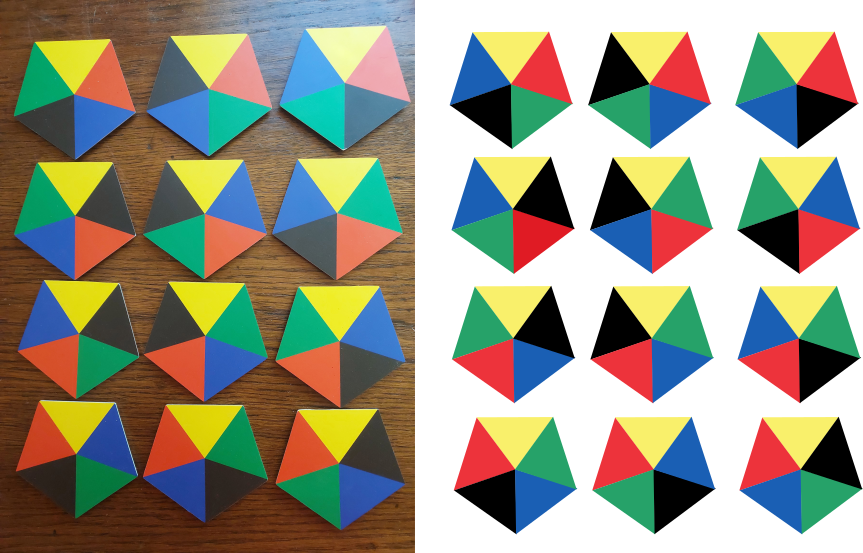
Les 12 cares on el que es demana és fer coincidir el color de les arestes. El repte de fer coincidir els vèrtexs seria similar amb una petita rotació.
Les 12 permutacions que no hem utilitzat i amb les que també podrien vestir el dodecaedre. S’han obtingut de les permutacions de l’esquerra intercanviant (transposant) el verd i el blau.
