The tiling lizards
Try to fit these tiles, reproduced from the lithography "Reptiles" by the admired artist Maurits Cornelis Escher.
- Hotel Area: Sala Pere Puig Adam
- Minimum age: from 5 years old.
- Required time: 5 minutes.
- Number of participants: One or more people
- Keywords: Escher, tiles,
- Taxonomy: GEOMETRY


Reptiles (1943) lithography by Maurits Cornelis Escher.
Escher's personality
Maurits Cornelis Escher (1898–1972) was a Dutch artist, famous for his drawings ofimpossible objects, representations of infinity, reflections, symmetries, perspectives, polyhedras, tesstellations and other mathematical objects. His admired works stand out for their realism achieved with a great technical command of light and perspective.
His work is inspired by nature, mosaics such as those of the Granada Shadow and mathematical objects resulting from his interaction with personalities such as George Pólya, Roger Penrose, Harold Coxeter or Martin Gardner.
Although today we recognize him as a great artist, this was not the case for most of his life. The first retrospective exhibition was held when she was 70 years old.
The transformation of hexagonal tiling
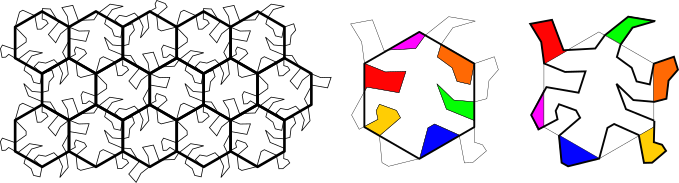
The tiles, or tiles in the form of lizards, are a modification of the hexagonal tiles that cover the plane.
The image shows how to crop six parts of a hexagon and
then incorporating them into the appropriate places to form the lizard.
Mmaca store lizards
In the MMACA store you can buy a box with 15 lizards accompanied by an explaining sheet and with proposals to create new Escher-shaped mosaics
Other modules in this room
The curves that are obtained by rolling circumferences of different sizes.
Look at the sections of objects illuminated by the red LEDs of this ring.
Try putting the tetraeder and octahedre in the cube.
Build polyhedras with magnetized pieces.
With all the pieces we build 3, 2 or 1 equilateral triangles
The artisanal wooden cone that shows its sections: Circumference, ellipse, parabola and hyperbola.
With the same pieces, it reconstructs two polygons.
Multiple ways to visualize and understand this famous theorem
Two balls, one low in a straight line, the other curving. What's the point before?
We raise these arches with pillows.
How do the lengths, surfaces and volumes of similar objects change?
Put the blue poles, perpendicular to the edges of the dodecaedre to build the icosaedre.
Displaying numeric properties with buckets and other pieces.